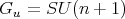Servicios Personalizados
Revista
Articulo
Indicadores
-
 Citado por SciELO
Citado por SciELO
Links relacionados
-
 Similares en
SciELO
Similares en
SciELO
Compartir
Revista de la Unión Matemática Argentina
versión impresa ISSN 0041-6932versión On-line ISSN 1669-9637
Rev. Unión Mat. Argent. v.47 n.1 Bahía Blanca ene./jun. 2006
On the Variety of Planar Normal Sections
Alicia N. García, Walter N. Dal Lago and Cristián U. Sánchez
Partially supported by SECYT-UNC and CONICET, Argentina
Abstract: In the present paper we present a survey of results concerning the variety ![X [M ]](/img/revistas/ruma/v47n1/1a110x.png) of planar normal sections associated to a natural embedding of a real flag manifold
of planar normal sections associated to a natural embedding of a real flag manifold  . The results included are those that, we feel, better describe the nature of this algebraic variety of
. The results included are those that, we feel, better describe the nature of this algebraic variety of  . In particular we present results concerning its Euler characteristic showing that it depends only on
. In particular we present results concerning its Euler characteristic showing that it depends only on  and not on the nature of
and not on the nature of  itself. Furthermore, when
itself. Furthermore, when  is the manifold of complete flags of a compact simple Lie group, we present what is, in some sense, its dimension and a large class of submanifolds of
is the manifold of complete flags of a compact simple Lie group, we present what is, in some sense, its dimension and a large class of submanifolds of  contained in
contained in ![X [M ]](/img/revistas/ruma/v47n1/1a117x.png) .
.
In Differential Geometry, the study of submanifolds is frequently associated to the to the theme of "normal sections". This was already present in works of Euler (1707-1783) when he studied surfaces embedded in 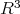 . Given a surface
. Given a surface 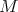 embedded in
embedded in 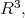 we can obtain geometric information about the surface itself and the way it is contained in
we can obtain geometric information about the surface itself and the way it is contained in  via properties of the curves that are obtained by cutting the surface with planes determined by unit tangent vectors and the normal vector to the surface at each point. This curves are called normal sections and they give information about the intrinsic and extrinsic geometry of the surface
via properties of the curves that are obtained by cutting the surface with planes determined by unit tangent vectors and the normal vector to the surface at each point. This curves are called normal sections and they give information about the intrinsic and extrinsic geometry of the surface 
Approximately in 1980, Bang Yen Chen generalized this notion for submanifolds of 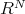 of codimension larger than 1, in the following natural manner:
of codimension larger than 1, in the following natural manner:
Let 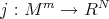 be an isometric immersion and
be an isometric immersion and 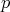 a point in
a point in 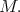 We identify a neighborhood of
We identify a neighborhood of  with its image by
with its image by 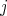 and consider, in the tangent space
and consider, in the tangent space  a unit vector
a unit vector  If
If 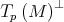 denotes the normal space to
denotes the normal space to 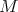 at
at 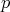 , we may define an affine subspace of
, we may define an affine subspace of 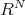 by
by
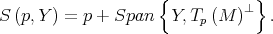
If 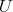 is a small enough neighborhood of
is a small enough neighborhood of 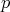 in
in 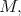 then the intersection
then the intersection  can be considered the image of a
can be considered the image of a  regular curve
regular curve 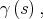 parametrized by arc-length, such that
parametrized by arc-length, such that 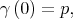
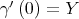 . This curve is called a normal section of
. This curve is called a normal section of 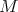 at p in the direction of
at p in the direction of 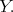 In a strict sense, we ought to speak of the "germ" of a normal section at
In a strict sense, we ought to speak of the "germ" of a normal section at 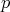 determined by the unit vector
determined by the unit vector 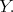 A change in the neighborhood
A change in the neighborhood 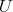 will change the curve; however, this new curve will coincide with
will change the curve; however, this new curve will coincide with 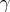 in a neighborhood of zero. Since our computations with the curve
in a neighborhood of zero. Since our computations with the curve 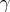 are done at the point
are done at the point  we may take any one of these curves. We may also assume that
we may take any one of these curves. We may also assume that 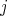 is an embedding.
is an embedding.
Since 1980, several authors, for instance Chen, Verheyen, Deprez ([2], [3], [8]) have studied geometric properties de submanifolds of Euclidean spaces in term of their normal sections. They obtained interesting results which characterize submanifolds of 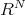 where: the geodesic are planar; the normal sections are geodesics; the normal sections have the same constant curvature; etc.
where: the geodesic are planar; the normal sections are geodesics; the normal sections have the same constant curvature; etc.
Following B.Y. Chen, we say that the normal section 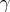 of
of 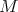 at
at 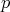 in the direction of
in the direction of 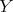 is pointwise planar at
is pointwise planar at 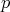 if its first three derivatives
if its first three derivatives 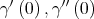 and
and 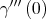 are linearly dependent, i.e. if
are linearly dependent, i.e. if 
In 1982, Chen obtained the following interesting result.
Theorem 1. [2] A spheric submanifold of 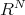 (i.e. contained in a sphere) has all its normal sections pointwise planar if and only if the second fundamental form is parallel.
(i.e. contained in a sphere) has all its normal sections pointwise planar if and only if the second fundamental form is parallel.
This fact was for us of the particular interest because, in 1980, Ferus had related symmetric R-spaces with properties of the second fundamental form through the following:
Theorem 2. [9] A spheric submanifold of 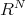 is a symmetric R-space if and only if the second fundamental form is parallel.
is a symmetric R-space if and only if the second fundamental form is parallel.
The previous theorems clearly yield the following:
Theorem 3. A spheric submanifold of 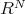 is a symmetric R-spaces if and only if all its normal sections are pointwise planar.
is a symmetric R-spaces if and only if all its normal sections are pointwise planar.
2. The variety of planar normal sections
As consequence of the Theorem 3, for a spheric submanifold  of
of 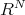 , the set of tangent vectors which define pointwise planar normal sections contains information about whether a given R-space is or not symmetric. So, if for each
, the set of tangent vectors which define pointwise planar normal sections contains information about whether a given R-space is or not symmetric. So, if for each 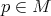 , we denote by
, we denote by ![^ Xp [M ]](/img/revistas/ruma/v47n1/1a1158x.png) the set of
the set of 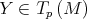 such that
such that 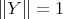 and define pointwise planar normal sections, we have that
and define pointwise planar normal sections, we have that  is a symmetric R-space if and only if
is a symmetric R-space if and only if ![X^p [M ] = Sm -1,](/img/revistas/ruma/v47n1/1a1162x.png)
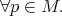 Therefore, if
Therefore, if  is a R-space which is not symmetric we have that
is a R-space which is not symmetric we have that ![X^p [M ] ⊊ Sm -1.](/img/revistas/ruma/v47n1/1a1165x.png)
Our first objective was to obtain information about ![^ Xp [M ]](/img/revistas/ruma/v47n1/1a1166x.png) when
when 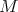 is a R-space (also called real flag manifold).
is a R-space (also called real flag manifold).
We recall that an R-space or a real flag manifold is an orbit of an s-representation. The reader is referred to [4, p. 225] and references therein, for basic information concerning R-spaces, canonical connections, etc.
For our study we need methods and techniques different from the known ones. The first result that we obtained in this direction was the following.
Theorem 4. [4, (2.5)] If 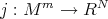 is a natural embedding of a real flag manifold and
is a natural embedding of a real flag manifold and 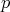 is a point in
is a point in 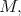 then the normal section
then the normal section 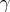 with
with  and
and  is pointwise planar at
is pointwise planar at 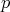 if and only if the unit tangent vector
if and only if the unit tangent vector 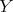 at
at 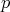 satisfies the equation
satisfies the equation
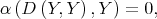
where 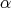 is the second fundamental form of the embedding
is the second fundamental form of the embedding 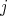 and
and  denotes the difference tensor between the Riemannian connection
denotes the difference tensor between the Riemannian connection  (associated to the metric induced from the Euclidean metric) and the canonical connection
(associated to the metric induced from the Euclidean metric) and the canonical connection 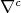 (associated to the "usual" reductive decomposition of the Lie algebra of the compact Lie group defining
(associated to the "usual" reductive decomposition of the Lie algebra of the compact Lie group defining 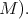
Then, given a point 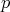 in the real flag manifold
in the real flag manifold 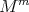
![^Xp [M ] = {Y ∈ Tp (M ) : ∥Y ∥ = 1,α (D (Y,Y ) ,Y) = 0}.](/img/revistas/ruma/v47n1/1a1186x.png)
Since ![Y ∈ X^p [M ]](/img/revistas/ruma/v47n1/1a1187x.png) clearly implies
clearly implies ![- Y ∈ X^p [M ],](/img/revistas/ruma/v47n1/1a1188x.png) we may take
we may take ![Xp [M ]](/img/revistas/ruma/v47n1/1a1189x.png) as the image of this set in the real projective space
as the image of this set in the real projective space 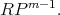 Since
Since  is an orbit of a group of isometries of the ambient space
is an orbit of a group of isometries of the ambient space 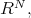 it is clear that
it is clear that ![Xp [M ]](/img/revistas/ruma/v47n1/1a1193x.png) does not depend on the point
does not depend on the point 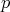 and we may denote it by
and we may denote it by ![X [M ].](/img/revistas/ruma/v47n1/1a1195x.png)
The last theorem allowed us to describe tangent vectors which define pointwise planar normal sections as solutions of an equation and furthermore to obtain the following interesting consequence.
Corollary 1. [4, (2.9)] ![X [M ]](/img/revistas/ruma/v47n1/1a1196x.png) is a real algebraic variety of
is a real algebraic variety of 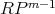 and its natural complexification
and its natural complexification ![Xc [M ]](/img/revistas/ruma/v47n1/1a1198x.png) is a complex algebraic variety of
is a complex algebraic variety of  , defined both by homogeneous polynomials of degree 3.
, defined both by homogeneous polynomials of degree 3.
These varieties measure, in some sense, how far is the real flag manifold 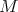 from being a symmetric space (i.e. a symmetric real flag manifold). By Theorem 3,
from being a symmetric space (i.e. a symmetric real flag manifold). By Theorem 3, ![X[M ]](/img/revistas/ruma/v47n1/1a11101x.png) and
and ![X [M ] c](/img/revistas/ruma/v47n1/1a11102x.png) differ respectively from
differ respectively from  and
and 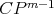 . However, surprisingly enough, they have the same Euler characteristic as we see in the following:
. However, surprisingly enough, they have the same Euler characteristic as we see in the following:
Theorem 5. [4], [14] Let  be a real flag manifold and let
be a real flag manifold and let 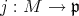 be its natural imbedding. Let
be its natural imbedding. Let ![m -1 X [M ] ⊂ RP](/img/revistas/ruma/v47n1/1a11107x.png) be the variety of directions of pointwise planar normal sections at a point
be the variety of directions of pointwise planar normal sections at a point 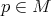 and let
and let ![Xc [M ] ⊂ CP m- 1](/img/revistas/ruma/v47n1/1a11109x.png) be the natural complexification of
be the natural complexification of ![X [M ]](/img/revistas/ruma/v47n1/1a11110x.png) . If
. If 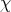 denotes the Euler characteristic with respect to rational coefficients, then
denotes the Euler characteristic with respect to rational coefficients, then
![{ (i) χ (X [M ]) = χ (RP m-1) = 0 if dim M is even 1 if dim M is odd (ii) χ (Xc [M ]) = χ (CP m-1) = m = dim M.](/img/revistas/ruma/v47n1/1a11112x.png)
In [4] we gave a proof of this fact when 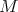 is a complex flag manifold. The methods used in that paper were not strong enough to tackle the general case. However, several years later we were able to obtain the proof for the general case (see [14]).
is a complex flag manifold. The methods used in that paper were not strong enough to tackle the general case. However, several years later we were able to obtain the proof for the general case (see [14]).
3. Submanifolds in the variety of planar normal sections
Looking for information about the "size" of ![X [M ],](/img/revistas/ruma/v47n1/1a11114x.png) we studied the existence of a great deal of smooth subvarieties embedded into
we studied the existence of a great deal of smooth subvarieties embedded into 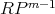 and contained in
and contained in ![X [M ],](/img/revistas/ruma/v47n1/1a11116x.png) when
when  is a manifold of complete flags of a compact simple Lie group.
is a manifold of complete flags of a compact simple Lie group.
In order to indicate our results we need introduce the following notation.
Let 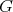 be a simply connected, complex, simple Lie group and let
be a simply connected, complex, simple Lie group and let  be its Lie algebra. Let
be its Lie algebra. Let  be a Cartan subalgebra of
be a Cartan subalgebra of 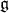 and
and 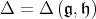 the root system of
the root system of 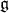 relative to
relative to  We may write
We may write 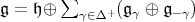 , where
, where  indicates the set of positive roots with respect to some order.
indicates the set of positive roots with respect to some order.
Let us consider in 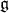 the Borel subalgebra
the Borel subalgebra 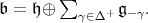 Let
Let 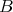 be the analytic subgroup of
be the analytic subgroup of 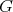 corresponding to the subalgebra
corresponding to the subalgebra 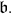
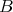 is closed and its own normalizer in
is closed and its own normalizer in 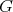 . The quotient space
. The quotient space  is a complex homogeneous space called the manifold of complete flags of
is a complex homogeneous space called the manifold of complete flags of 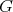 .
.
Let 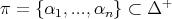 be a system of simple roots. We may take in
be a system of simple roots. We may take in 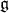 a Weyl basis [12, III, 5]
a Weyl basis [12, III, 5] 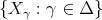 and
and 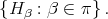 The following set of vectors provides a basis of a compact real form
The following set of vectors provides a basis of a compact real form 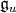 of
of  .
.
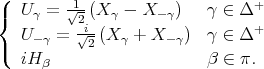 | (1) |
We shall denote by 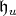 the real vector space generated by
the real vector space generated by 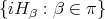 and by
and by 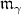 that of
that of  Then we may write
Then we may write 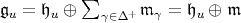 .
.
Let  be the analytic subgroup of
be the analytic subgroup of  corresponding to
corresponding to 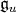 .
.  is compact and acts transitively on
is compact and acts transitively on  which can be written as
which can be written as 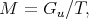 where the subgroup
where the subgroup 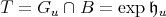 is a maximal torus in
is a maximal torus in 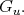 The manifold
The manifold 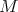 is then a compact simply connected complex manifold. This is the manifold of complete flags for the given compact connected simple Lie group
is then a compact simply connected complex manifold. This is the manifold of complete flags for the given compact connected simple Lie group  In the rest of this paper we shall restrict our attention to this case.
In the rest of this paper we shall restrict our attention to this case.
It is well known that 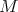 is the orbit of a regular element
is the orbit of a regular element 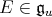 by the adjoint action of
by the adjoint action of 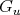 on
on 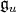 . Then we have a natural embedding
. Then we have a natural embedding 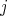 of
of  on
on 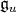 which we may assume isometric by taking in
which we may assume isometric by taking in 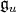 the inner product given by the opposite of the Killing form.
the inner product given by the opposite of the Killing form.
Then the tangent and normal space to 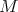 at
at 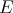 are
are ![TE (M ) = [𝔤u,E] = [𝔪, E] = 𝔪](/img/revistas/ruma/v47n1/1a11168x.png) and
and 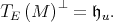
If 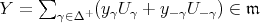 then
then ![-- Y = [Y, E] ∈ TE (M )](/img/revistas/ruma/v47n1/1a11171x.png) and for the second fundamental form of the embedding
and for the second fundamental form of the embedding 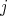 we may write
we may write
![∑ α ([Y, E] ,D ([Y, E] ,[Y, E])) = [Y, [Y, [Y,E]]𝔪]𝔥u = 1≤r≤npriH γr.](/img/revistas/ruma/v47n1/1a11173x.png) | (2) |
The coefficients  are homogeneous polynomials of degree
are homogeneous polynomials of degree 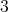 in the variables
in the variables 
 Then, by Theorem 4,
Then, by Theorem 4, 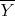 defines a pointwise planar normal section if and only if
defines a pointwise planar normal section if and only if  for
for 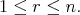
3.1. Fat Submanifold. We obtained explicit enough expressions for the polynomials 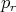 defined by (2), which allowed us to prove that they are
defined by (2), which allowed us to prove that they are 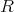 -linearly dependent but this is not the case for any subset of them with
-linearly dependent but this is not the case for any subset of them with  elements.
elements.
Theorem 6. [7] The polynomials 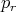 (
( ) defined in (2) satisfy:
) defined in (2) satisfy:
(i)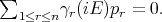
(ii) For any 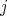 such that
such that 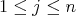 the set
the set 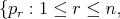
 is
is  linearly independent.
linearly independent.
With this fact, we can get certain information about the size of ![X [M ].](/img/revistas/ruma/v47n1/1a11192x.png)
Theorem 7. [7] There is an open set in the variety ![X [M ]](/img/revistas/ruma/v47n1/1a11193x.png) which is an embedded submanifold in
which is an embedded submanifold in 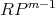 of dimension
of dimension  where
where 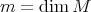 and
and 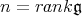 .
.
(The topology of ![X[M ]](/img/revistas/ruma/v47n1/1a11198x.png) is the induced one from the usual topology of
is the induced one from the usual topology of 
To get this result it was necessary to find points 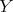 in
in 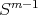 , the unit sphere of
, the unit sphere of  , such that they are regular points of the function
, such that they are regular points of the function 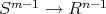 whose coordinates are the polynomials
whose coordinates are the polynomials  and that satisfy
and that satisfy ![[Y ] ∈ X[M ].](/img/revistas/ruma/v47n1/1a11205x.png)
3.2. Projective subspaces in ![X[M ]](/img/revistas/ruma/v47n1/1a11206x.png) . Another way to get information about the "size" of
. Another way to get information about the "size" of ![X [M ]](/img/revistas/ruma/v47n1/1a11207x.png) is to know a sufficient amount of projective subspaces in it.
is to know a sufficient amount of projective subspaces in it.
We shall denote by 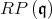 the real projective space associated to a real vector space
the real projective space associated to a real vector space 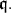
Associated to the simple group 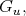 defining the complex flag manifold
defining the complex flag manifold 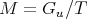 , we have its family of symmetric spaces of type I [12, p. 518] and among them, we want to consider those which are inner, i.e. the spaces in which the symmetry at each point belongs to the group
, we have its family of symmetric spaces of type I [12, p. 518] and among them, we want to consider those which are inner, i.e. the spaces in which the symmetry at each point belongs to the group 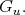 Among all compact symmetric spaces, these are the only ones strongly related with the algebraic variety
Among all compact symmetric spaces, these are the only ones strongly related with the algebraic variety ![X [M ].](/img/revistas/ruma/v47n1/1a11213x.png) It is well known that each one of the simple groups gives rise to at least one of these symmetric spaces. They are those of the form
It is well known that each one of the simple groups gives rise to at least one of these symmetric spaces. They are those of the form  where
where 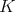 is a subgroup of maximal rank in
is a subgroup of maximal rank in 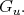 The ones which are not inner in the list in [12, p. 518] are
The ones which are not inner in the list in [12, p. 518] are 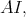
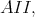
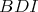
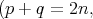

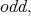
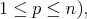
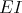 and
and 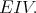
By conjugating 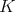 if necessary, we may assume that
if necessary, we may assume that 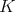 contains
contains 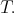
Let 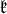 be the Lie algebra of
be the Lie algebra of 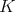 and write
and write 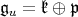 where
where 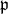 is the orthogonal complement to
is the orthogonal complement to 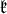 with respect to the Killing form. Then
with respect to the Killing form. Then 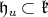 and
and 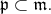
The motivation to consider the tangent space 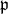 to the inner symmetric space
to the inner symmetric space 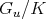 , in our study of the algebraic variety
, in our study of the algebraic variety ![X [M ],](/img/revistas/ruma/v47n1/1a11238x.png) arises from the following simple fact which provides the first examples of projective subspaces included in
arises from the following simple fact which provides the first examples of projective subspaces included in ![X [M ]](/img/revistas/ruma/v47n1/1a11239x.png) .
.
Proposition 1. [5, Prop. 4.1] Let  be the tangent space of the inner symmetric space
be the tangent space of the inner symmetric space 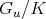 at
at ![[K] .](/img/revistas/ruma/v47n1/1a11242x.png) Then
Then ![RP (𝔭) ⊂ X [M ].](/img/revistas/ruma/v47n1/1a11243x.png)
Remark 1. [5, Rem. 4.1] For the subspace 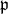 mentioned in the last proposition, there exists a root
mentioned in the last proposition, there exists a root 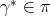 such that
such that 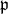 is of the form
is of the form
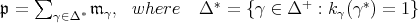
and 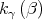 is defined by
is defined by 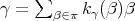 .
.
For this, it was natural to start by studying those subspaces of the tangent space to  at
at ![o = [T ]](/img/revistas/ruma/v47n1/1a11251x.png) of the form
of the form

and such that ![( ) RP ^𝔭 ⊂ X [M ].](/img/revistas/ruma/v47n1/1a11253x.png)
The subspaces 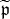 mentioned above, are exactly those subspaces of the tangent space
mentioned above, are exactly those subspaces of the tangent space 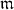 which are
which are  -invariant (see for instance [11]).
-invariant (see for instance [11]).
The first important result to our objective, was the following characterization, in terms of the structure of the Lie algebra of the simple group  This characterization was a very useful tool for the proof of many of the results obtained.
This characterization was a very useful tool for the proof of many of the results obtained.
Theorem 8. [5, Th. 4.2] Set 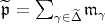 with
with 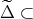
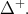 Then
Then
![( ) RP (^𝔭) ⊂ X [M ] ⇐ ⇒ ɛ, ρ ∈ ^Δ ⇒ ɛ + ρ ∕∈ ^Δ .](/img/revistas/ruma/v47n1/1a11261x.png)
The tangent spaces of the inner symmetric space associated to 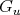 play an important part among the subspaces
play an important part among the subspaces 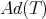 -invariant of the tangent space
-invariant of the tangent space 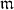 . This can be seen in the following two theorems.
. This can be seen in the following two theorems.
Theorem 9. [5, Th. 4.3] Let 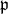 be the tangent space of the inner symmetric space
be the tangent space of the inner symmetric space 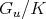 at
at ![[K] .](/img/revistas/ruma/v47n1/1a11267x.png) Then
Then 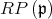 is maximal among the projective spaces
is maximal among the projective spaces 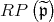 contained in
contained in ![X [M ],](/img/revistas/ruma/v47n1/1a11270x.png) with
with 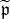 of the form
of the form  for
for 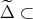
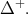
This theorem is the best we can hope to get for projective subspaces ![RP (𝔭) ⊂ X [M ]](/img/revistas/ruma/v47n1/1a11275x.png) arising from tangent spaces
arising from tangent spaces 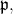 at the base point
at the base point ![[K]](/img/revistas/ruma/v47n1/1a11277x.png) , of irreducible inner symmetric spaces
, of irreducible inner symmetric spaces 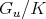 . We were able to show that if
. We were able to show that if  the projective spaces generated by those
the projective spaces generated by those  are not maximal among all the projective spaces contained in
are not maximal among all the projective spaces contained in ![X [M ]](/img/revistas/ruma/v47n1/1a11281x.png) (see [5, section 5]).
(see [5, section 5]).
The irreducible inner symmetric spaces 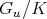 for which
for which 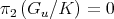 , are the following
, are the following
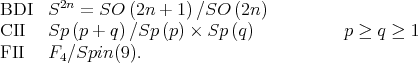
These are those whose tangent spaces, at the basic point, are of the form
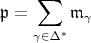
where  is the set of all short roots. We proved that for the spaces of the families BDI, CII and the single space FII, the tangent space
is the set of all short roots. We proved that for the spaces of the families BDI, CII and the single space FII, the tangent space 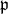 does not generate a maximal projective space in
does not generate a maximal projective space in ![X[M ].](/img/revistas/ruma/v47n1/1a11288x.png) However these are the only ones with this property as the following result indicates.
However these are the only ones with this property as the following result indicates.
Theorem 10. [5, Th. 4.4] Let 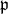 be the tangent space of the inner symmetric space
be the tangent space of the inner symmetric space 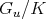 at
at ![[K] .](/img/revistas/ruma/v47n1/1a11291x.png) Then
Then 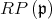 is maximal in
is maximal in ![X [M ]](/img/revistas/ruma/v47n1/1a11293x.png) if and only if
if and only if  does not vanish.
does not vanish.
Another question arises quite naturally. How large can a subspace  -invariant defining projective spaces contained in
-invariant defining projective spaces contained in ![X [M ]](/img/revistas/ruma/v47n1/1a11296x.png) be?. Clearly an answer to this question yields information about the "size" of the variety
be?. Clearly an answer to this question yields information about the "size" of the variety ![X [M ].](/img/revistas/ruma/v47n1/1a11297x.png)
The tangent spaces of the irreducible symmetric spaces are deeply related to this question and, as we expected, they provide the  -invariant subspaces
-invariant subspaces 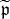 of larger dimension such that
of larger dimension such that 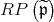 is contained in the variety of planar normal sections.
is contained in the variety of planar normal sections.
The list of irreducible symmetric spaces [12, p. 518] indicates that the irreducible inner symmetric spaces of maximal dimension for given groups  are those included in the following table with their respective dimensions. We denote them by
are those included in the following table with their respective dimensions. We denote them by 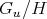 and
and 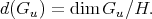

Theorem 11. [6, Th. 1.1, Th. 1.2] If 
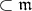 is a subspace
is a subspace 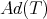 -invariant defining a projective subspace in
-invariant defining a projective subspace in ![X[Gu ∕T ]](/img/revistas/ruma/v47n1/1a11308x.png) , then
, then
(i) 
(ii) If 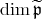
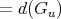 then
then 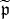 is tangent to the symmetric space
is tangent to the symmetric space 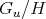 at a fixed point of the action of the torus
at a fixed point of the action of the torus 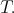
The existence of projective subspaces in the variety of planar normal sections makes it rather special.
In the previous section we gave information about families of projective subspaces in ![X[Gu ∕T]](/img/revistas/ruma/v47n1/1a11316x.png) which have deep relation with the tangent spaces of the inner symmetric spaces associated to the simple group
which have deep relation with the tangent spaces of the inner symmetric spaces associated to the simple group 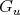 . This subspaces originate in some
. This subspaces originate in some 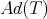 - invariant subspaces of the tangent space of
- invariant subspaces of the tangent space of 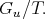
In [11], related to the study of extrinsic symmetric CR-structures on the manifold of complete flags 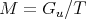 , it was observed that there is a strong connection between the holomorphic tangent spaces of these structures and those subspaces of the tangent space to
, it was observed that there is a strong connection between the holomorphic tangent spaces of these structures and those subspaces of the tangent space to 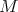 which are
which are 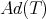 - invariant and also give rise to projective subspaces in
- invariant and also give rise to projective subspaces in ![X [M ]](/img/revistas/ruma/v47n1/1a11323x.png) . This particular fact throws new light on the interest of the study of these subspaces in
. This particular fact throws new light on the interest of the study of these subspaces in ![X [M ]](/img/revistas/ruma/v47n1/1a11324x.png) .
.
In the previous theorems we characterize, for the manifolds of the form  , those subspaces
, those subspaces  of the tangent space to
of the tangent space to 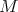 which are
which are 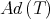 -invariant and define projective subspaces of maximal dimension in
-invariant and define projective subspaces of maximal dimension in ![X [M ]](/img/revistas/ruma/v47n1/1a11329x.png) . Making a deeper analysis in this direction, for the manifolds
. Making a deeper analysis in this direction, for the manifolds 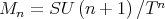 , we continued studying those subspaces
, we continued studying those subspaces 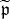 that are
that are 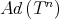 -invariant and define projective subspaces in
-invariant and define projective subspaces in ![X [Mn]](/img/revistas/ruma/v47n1/1a11333x.png) but that, in some "interesting" sense, are of "minimal" dimension. These subspaces are those
but that, in some "interesting" sense, are of "minimal" dimension. These subspaces are those 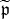 , which are of minimal dimension and not properly contained in any other
, which are of minimal dimension and not properly contained in any other 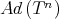 -invariant subspace, defining projective subspaces in
-invariant subspace, defining projective subspaces in ![X [Mn]](/img/revistas/ruma/v47n1/1a11336x.png) . For these subspaces we have obtained the following:
. For these subspaces we have obtained the following:
Theorem 12. [7] Let 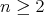 and
and 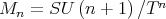 be embedded in
be embedded in 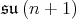 as the orbit of any regular element
as the orbit of any regular element 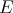 . Let
. Let 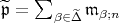 (
( ) be a subspace of
) be a subspace of  which is maximal among the subspaces
which is maximal among the subspaces  -invariant of
-invariant of 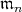 defining projective subspaces in
defining projective subspaces in ![X [Mn] .](/img/revistas/ruma/v47n1/1a11346x.png) Then
Then
(i) 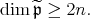
(ii) If 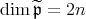 then
then 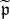 is the tangent space to the projective space
is the tangent space to the projective space  at a point
at a point 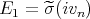 where
where  is defined in [15, p. 80] and
is defined in [15, p. 80] and 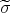 is an element in
is an element in 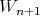 , the Weyl group of the pair
, the Weyl group of the pair 
Due to the fact that the converse statement of (ii) above is obviously true, this theorem gives a geometric characterization of the subspaces 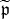 of
of  which are
which are  -dimensional, defining projective subspaces in
-dimensional, defining projective subspaces in ![X [Mn]](/img/revistas/ruma/v47n1/1a11359x.png) and maximal among the subspaces
and maximal among the subspaces 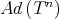 -invariant of
-invariant of 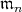 .
.
Joining Theorems 11 and 12, the subspaces 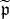 of
of 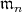 which are maximal among the subspaces
which are maximal among the subspaces 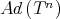 -invariant of
-invariant of 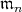 defining projective subspaces in
defining projective subspaces in ![X [Mn]](/img/revistas/ruma/v47n1/1a11366x.png) , satisfy
, satisfy

and also, when 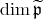 is one of the two ends of the above inequality, the subspace
is one of the two ends of the above inequality, the subspace 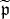 is tangent to the inner symmetric space of minimal and maximal dimension associated to the group
is tangent to the inner symmetric space of minimal and maximal dimension associated to the group 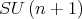 .
.
When the subspace 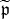 is such that
is such that 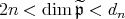 , if we pose no restriction on
, if we pose no restriction on 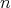 and
and  we cannot assure that
we cannot assure that 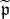 is tangent to some inner symmetric space of the group
is tangent to some inner symmetric space of the group 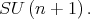 Furthermore, we give examples in [7] to show that we cannot even assure that
Furthermore, we give examples in [7] to show that we cannot even assure that 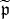 is tangent to a homogeneous manifold
is tangent to a homogeneous manifold  with
with 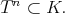
The obtained results allow us to mention the following consequences which we feel are interesting and that in some sense motivated our interest in having a deeper understanding of the projective subspaces in the variety of planar normal section.
Keeping the notation of [11, Th.8] and calling
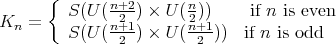
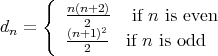
we may write :
Corollary 2. [7] Let 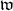 be maximal among the holomorphic tangent spaces at the base point of
be maximal among the holomorphic tangent spaces at the base point of 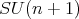 -invariant minimal almost Hermitian extrinsic symmetric CR-structure on
-invariant minimal almost Hermitian extrinsic symmetric CR-structure on 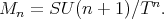 Then
Then
(i) 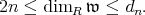
(ii) If  or
or 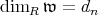 then
then 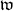 is the tangent space, at some point, to the projective space
is the tangent space, at some point, to the projective space  or the symmetric space
or the symmetric space  respectively.
respectively.
[1] Burstall F. E. - Rawnsley J. H. Twistor theory for Riemannian Symmetric Spaces Lecture Notes in Math. 1424. Springer Verlag 1995. [ Links ]
[2] Chen, B. Y.: Differential geometry of submanifolds. with planar normal sections, Ann. Mat. Pura Appl. 130 (1982), 59-66. [ Links ]
[3] Chen, B. Y. and Verheyen, P.: Sous-variétés dont les sectiones normales son des géodésiques, C.R. Acad. Sci. Paris Ser A 293 (1981), 611-613. [ Links ]
[4] Dal Lago, W. , García, A. and Sánchez, C.: Planar normal sections on the natural imbedding of a flag manifold, Geom. Dedicata 53 (1994), 223-235. [ Links ]
[5] Dal Lago, W. , García, A. and Sánchez, C.:Maximal projective subspaces in the variety of planar normal sections of a flag manifold, Geom. Dedicata 75 (1999), 219-233. [ Links ]
[6] Dal Lago, W. , García, A. and Sánchez, C.:Projective subspaces in the variety of normal sections and tangent spaces to a symmetric space, J. of Lie Theory 8 (1998), 415-428. [ Links ]
[7] Dal Lago, W. , García, A. and Sánchez, C.:Submanifolds in the variety of normal sections, Beiträge zur Algebra und Geometrie 47 (2006), 289-304. [ Links ]
[8] Deprez, J. and Verheyen, P.: Immersions with circular normal sections and normal sections of product immersions, Geom. Dedicata 20 (1986), 335-344. [ Links ]
[9] Ferus, D.: Symmetric submanifolds of Euclidean spaces, Math. Ann. 247 (1980), 81-93. [ Links ]
[10] Ferus, D.:Immersionen mit paralleler zweiter Fundamentalform: Beispiele und Nicht-Beispiele, Manuscripta math. 12, (1974), 153-162. [ Links ]
[11] García, A. and Sánchez, C.: On extrinsic symmetric CR-structures on the manifolds of complete flags. Beiträge zur Algebra und Geometrie 45 (2004), 401-414. [ Links ]
[12] Helgason, S.: Differential Geometry, Lie Groups and Symmetric Spaces, Academic Press, 1978. [ Links ]
[13] Humphreys J. E. Introduction to Lie Algebras and Representation Theory, Springer-Verlag Berlin. Heidelberg. New York 1972. [ Links ]
[14] Sánchez, C., García, A. and Dal Lago, W.: Planar normal sections on the natural imbedding of a real flag manifold. Beiträge zur Algebra und Geometrie 41 (2000), 513-530. [ Links ]
[15] Wolf, J. and Gray, A. Homogeneous spaces defined by Lie group automorphisms. I. J. Differential Geometry 2 (1968),77-114. [ Links ]
Alicia N. García
FaMAF-CIEM (UNC-CONICET)
Ciudad Universitaria
5000 Córdoba - Argentina.
agarcia@mate.uncor.edu
Walter N. Dal Lago
FaMAF-CIEM (UNC-CONICET)
Ciudad Universitaria
5000 Córdoba - Argentina.
dallago@mate.uncor.edu
Cristián U. Sánchez
FaMAF-CIEM (UNC-CONICET)
Ciudad Universitaria
5000 Córdoba - Argentina.
csanchez@mate.uncor.edu
Recibido: 24 de octubre de 2005
Aceptado: 3 de octubre de 2006