ARTÍCULOS

DOI: http://dx.doi.org/10.4279/PIP.090003

Self-sustained oscillations with delayed velocity feedback

 

D. H. Zanette¹*

*E-mail: zanette@cab.cnea.gov.ar

1 Centro Atómico Bariloche and Instituto Balseiro (Comisión Nacional de Energía Atómica, Universidad Nacional de Cuyo), Consejo Nacional de Investigaciones Científicas y Técnicas, Avda. E. Bustillo 9500, 8400 San Carlos de Bariloche, Río Negro, Argentina.

---

We study a model for a nonlinear mechanical oscillator, relevant to the dynamics of micro-and nanomechanical time-keeping devices, where periodic motion is sustained by a feedback force proportional to the oscillation velocity. Specifically, we focus our attention on the effect of a time delay in the feedback loop, assumed to originate in the electric circuit that creates and injects the self-sustaining force. Stationary oscillating solutions to the equation of motion, whose stability is insured by the crucial role of nonlinearity, are analytically obtained through suitable approximations. We show that a delay within the order of the oscillation period can suppress self-sustained oscillations. Numerical solutions are used to validate the analytical approximations.

Keywords Nonlinear oscillator; Self-sustained oscillation; Oscillation suppression; Micromechanical devices

---

 

I. Introduction

Inside any modern time-keeping device, the principal component is an oscillator which autonomously generates a stationary periodic signal with a well-defined frequency. The only external input to the system is the power needed to sustain the oscilla-tions. In devices based on mechanical oscillators -which comprise essentially all present-day clocks, with the exception of the atomic kind-, sustained periodic motion is achieved by forcing the oscillator with a conditioned version of the signal generated by the oscillator itself [1]. If this reinjected force is in-phase with the oscillation velocity, the resonant response is maximal, thus optimizing power con-sumption during the process of signal conditioning. Purely mechanical devices employed this feed-back scheme already in the Middle Ages. In fact, the escapement mechanism was routinely used in clock building since the 13th century. In modern clocks, oscillators are built from synthetic quartz crystals and feedback is implemented electronically. At the micro- and nanoscale, quartz crystals are expected to be replaced by simpler mechanical os-cillators such as tiny vibrating silica beams [2, 3], which are easily built during circuit printing and can be actuated by very small electric fields [4,5].

In a series of recent experiments on microme-chanical oscillators, it has been shown that self-sustained oscillations can be achieved with a feed-back force proportional to the oscillation velocity [6]. Since a purely linear mechanical system can-not display stable periodic motion, this kind of feedback force must necessarily be compensated by some nonlinear contribution from the oscillator dynamics itself. In the experiments, this balance turned out to come from the damping force, which was proportional to the velocity but showed a non-linear dependence on the oscillation amplitude.

In the present paper, we analyze a model for self-sustained periodic motion in a mechanical oscillator subjected to a feedback force proportional to the velocity, and nonlinear amplitude-dependent terms both in damping and in the restoring force. Emphasis is put on the effects of time delays in the feedback circuit, which modify the phase shift between the feedback forcé and the velocity. It is shown that these delays affect the response of the oscillator, to the point that stable periodic motion can even be suppressed. The model is studied analytically within suitable approximations, and the results are compared with numerical solutions to the equation of motion.

II. Mechanical model for self-sustained oscillations

Our model is based on an equation of motion for a one-dimensional variable x(t), which represents the departure from equilibrium of a mechanical oscillator:

where ¡i is the damping coefñcient per unit mass. The coefficients a and ¡3 weight the amplitude-dependent nonlinear corrections to the damping forcé and to the elastic forcé, respectively. The for-mer is a Van der Pol-like nonlinearity, while the cubic contribution to the restoring forcé defines a Duffing oscillator [7]. Both kinds of nonlinearity, with a, ¡3 > 0, have been experimentally verified to occur in micromechanical oscillators formed by silica beams clamped at their two ends (clamped-clamped, or c-c beams [4,6]). In the main oscilla-tion mode, c-c beams vibrate much like a plucked string, so that x(t) can be associated with the dis-placement of the middle point of the beam with respect to its rest position.

The right-hand side of Eq. (1) represents the feedback forcé per unit mass, which is proportional to the velocity at the delayed time t - t. As stated in the introduction, this delay is expected to originate in the electric circuit that reads, condi-tions, and reinjects the oscillator signal, due to the time elapsed during signal processing. Although t should be a very short time, it is not necessarily negligible as compared with other time scales in the system, in particular with the oscillation period. In fact, micro- and nano-oscillators vibrate with fre-quencies from the order of 100 kHz [4] to 1 GHz [8].

Time units in Eq. (1) have been chosen in such a way that the natural frequency of the undamped (p = 0), linear (J3 = 0), unforced (g = 0) oscillator equals unity. Meanwhile, the units of x can be fixed in such a way that the coefficient a adopts any prescribed valué. Henee, without generality loss and for future convenience, we fix a = 4.

i. Approximate stationary solutions

Nonlinearity in Eq. (1) prevenís obtaining an ex-act solution. Approximate stationary solutions can be found by the standard procedure of neglect-ing higher-harmonic contributions to the oscillations [7]. Within this approximation, and propos-ing x(t) = 7¡Aexr>(icüt) + c.c, we get a complex algebraic equation whose real and imaginary parts read

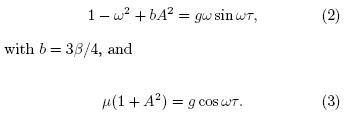
These are equations for the unknowns A and w, which have been obtained assuming A,u> =¿ 0.

Note that, to have a non-negative solution for A², Eq. (3) requires that g > ¡i. Physically, this amounts to require that the energy input from the feedback forcé is not less than the energy dissipated by damping. Otherwise, stationary periodic motion cannot be sustained. In the following, thus, we work under the assumption that such condition holds.

Squaring and summing up Eqs. (2) and (3), we obtain

namely, a relation between the oscillation ampli-tude A and the frequency uj which does not involve explicitly the delay t. Black (full and dotted) lines in Fig. 1 represent this relation for the parameters indicated in the figure.

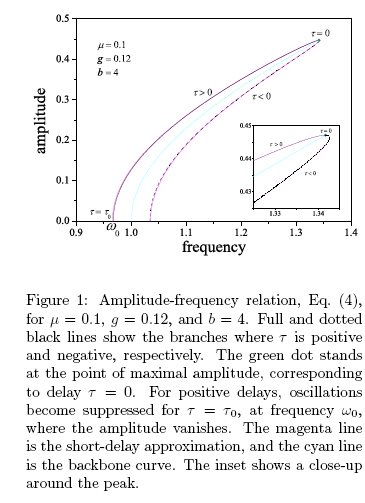
The graph of the relation between amplitude and frequency expressed by Eq. (4) can be interpreted as a resonance curve, in the sense that it characterizes the response of the oscillator to the feedback forcé. In fact, its shape resembles the upper part of the Dufíing resonance curve [7], and its mathe-matical origin is similar. From the physical point of view, however, it is important to stress that u> is not a control parameter -as in the standard case of an externally forced system- but emerges as an au-tonomous dynamical property of the self-sustained oscillator. The parameter whose variation defines the resonance curve of Fig. 1 is, on the other hand, the time delay t. In the figure, we have highlighted the point corresponding to t = 0 which, as can be seen from Eq. (3), corresponds to the máximum of the amplitude,

 

Different line types (full and dotted) in Fig. 1 rep-resent the branches of positive and negative t. Nat-urally, in an experiment, only the branch of positive time delays can be observed. However, stationary oscillatory solutions exist -and, as we discuss later, are stable- irrespectively of the sign of t.

ii. Oscillation suppression

The most relevant dynamical feature emerging from Eqs. (2) and (3) is that, as t varíes from zero to both positive and negative valúes, the oscillation amplitude decreases and, eventually, vanishes for sufiiciently long delays. This situation is reached at the points where the resonance curve in Fig. 1 in-tersects the horizontal axis. Focusing on the branch of positive delays, oscillations are suppressed when their frequency reaches

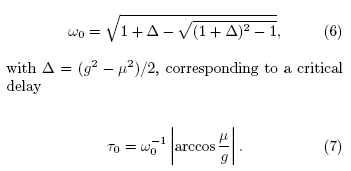
Oscillation suppression can be understood in qualitative terms as a consequence of the increas-ing phase shift between the feedback forcé and the velocity when t grows. As the phase shift becomes larger, the "timing" of energy supply by feedback fails to counteract energy dissipation by damping. The energy balance -implicit in Eq. (3)- cannot be further maintained, and periodic motion dies out.

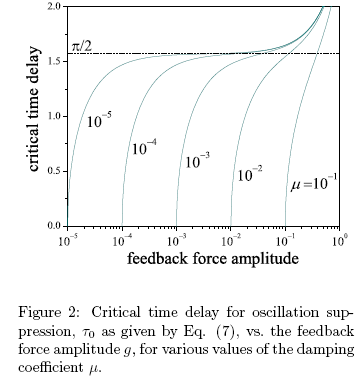
Figure 2 shows the critical delay tq at which oscillations are suppressed, as a function of the feedback amplitude g, and for various valúes of the damping ¡jl. For g just above /x, tq grows from zero and -if ¡jl is small enough- attains a plateau around n/2. Note that, since the oscillation frequency is always near unity, in this plateau the phase shift between feedback and velocity is also cióse to n/2. In other words, in this zone the feedback forcé is in-phase with the displacement x(t). For larger valúes of g, tq leaves the plateau and grows further.

We point out that very small valúes of the damping /x, as considered in Fig. 2, are realistic when feedback forcé amplitude working with micro- and nanomechanical oscilla-tors. Indeed, these devices have quality factors Q (~ M^_1) typically above 10⁴ [4]. In Fig. 1, on the other hand, we have chosen a relatively large valué of ¡jl for clarity in the graphical representation. For very small valúes of /x, in fact, the resonance curve becomes too narrow for its features to be clearly discerned in a plot.

iii. Stability of self-sustained oscillations

Stability of the stationary oscillatory solutions pre-sented above can be assessed by the method of múltiple time scales [7], which provides equations of motion for the relatively slow dynamics of the oscillation amplitude and phase. The method works under the assumption that there is a clear separa-tion between the oscillation period and other time scales involved in the system. In the present case, this condition is fulfilled for small damping, fil, as it is energy dissipation which controls the relax-ation of amplitude and frequency to their asymptotic valúes.

Application of the method of múltiple scales to Eq. (1) straightforwardly shows that when oscillatory solutions of non-vanishing amplitude do exist, they are stable under arbitrary perturbations to their amplitude and phase (or frequency), irrespectively of the sign of the delay. Instead of giving the details of this standard calculation, we resort here to a simple physical argument to explain stability, which sheds useful light on the role of nonlinearity.

Assume that the oscillator is in stationary peri-odic motion. This implies, in particular, that the energy input from feedback is exactly balanced by dissipation. If motion is now perturbed in such a way that the oscillation amplitude increases, the velocity increases accordingly, thus making feedback and damping to grow in the same proportion (since both are proportional to the velocity). However, due to the amplitude-dependent nonlinear correction in the damping coemcient, there is an extra growth in damping which enhances dissipation and therefore counteracts the perturbation, as the amplitude will tend to decrease. A symmetric argument applies if, on the contrary, the amplitude is perturbed to lower valúes.

If, on the other hand, the perturbation makes the frequency to increase, the velocity also increases as so do feedback and damping. Through the cubic nonlinearity in the restoring forcé, however, such change in the frequency leads the amplitude to grow. This growth, in turn, implies an enhance-ment in dissipation, as explained in the preceding paragraph. Consequently, the perturbation is coun-teracted by the oscillator response. The case where the frequency decreases is analogous. Under perturbations of both amplitude and frequency, therefore, nonlinearity plays a key role in insuring the stability of stationary oscillations.

III. Further approximations

i. Backbone approximation

As mentioned in section Il.ii., a realistic limit when working with micro- and nanomechanical oscilla-tors consists in considering very small valúes of the damping coemcient ¡i. In order to insure the ex-istence of physically meaningful solutions for the amplitude and the frequency, however, Eq. (3) re-quires that the limit of ¡i - 0 is taken along with the limit g - 0, maintaining a finite ratio r = g¡¡i. In physical terms, this joint limit is justified by the fact that the smaller the rate of energy dissipation, the smaller the feedback forcé necessary to maintain oscillatory motion.

 

Although, for the relatively large valué of ¡i con-sidered in Fig. 1, the backbone curve gives a poor approximation to the resonance curve; as ¡i be-comes smaller the two branches of the latter col-lapse to the backbone. A measure of this collapse is provided by computing the frequeney of oscilla-tion suppression, ljq, in the limit of small damping. Equation (6) yields

showing that the width of the resonance curve at its base (A = 0) is proportional to ¡i. In this limit, the time delay for oscillation suppression is still given by Eq. (7).

ii. Short-delay approximation

Another relevant approximation, which drastically simplifies the problem of solving Eqs. (2) and (3), is obtained for small valúes of t. In fact, approxi-mating síiícot « ujt and coswt k, 1 - uj²t²/2, we obtain linear equations for A² and cu², whose solu-tions yield

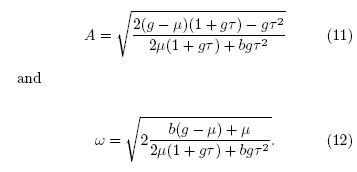
The magenta line in Fig. 1 represents these results. For the parameters of the figure, thus, this is an excellent approximation along the entire resonance curve.

IV. Numerical results

As a validation of the analytical results obtained by means of the approximation considered in sec-tion II., i.e., neglecting the higher-harmonic contri-butions to oscillatory motion, we have numerically solved Eq. (1) for various parameter sets. We have used a fourth-order Runge-Kutta scheme, with the only non-standard feature that, due to the delay in the feedback forcé, it is necessary to specify the solution x(t) for all times -t t 0, instead of the initial condition at t = 0. In all cases, we have considered a constant valué of x(t), henee with x(t) = 0, in that interval.

For all the parameter sets taken into account in the numerical calculations, we have found that, af-ter a transient time which scaled as yU,^-1, the os-cillator reached periodic motion. This observation is in qualitative agreement with our argument of section Il.iii., which prediets stability of periodic motion under very general conditions.

A more quantitative comparison between theo-retical and numerical results, illustrated for the parameter set of Fig. 1, is given in Fig. 3. The main panel shows, as green dots, numerical measure-ments of the frequeney and amplitude of long-time periodic motion for various (positive) valúes of the delay in the interval 0 < t < 0.4. The black full line stands for the theoretical result. The inset shows the same data in the amplitude-delay plañe. We see that the agreement is generally very good. Not un-expectedly, the theoretical approximation improves towards smaller amplitudes, where nonlinear effeets are weaker and periodic motion is better described by a puré harmonio oscillation, as assumed in section II. As a matter of fact, Fig. 3 is limited to amplitudes above 0.25 since, in the plot, theoretical and numerical results are indistinguishable for smaller valúes.

The agreement between our theoretical and numerical results rises the question to what extent higher-harmonic components, which are a direct product of nonlinearity but have been neglected in our analytical approximation, constitute a substantial contribution to oscillatory motion. To evalúate this, we calcúlate the amplitudes ofdifferent Fourier components in the long-time numerical so-lution for x(t). With the parameters of Fig. 3 and t = 0.1, for which the oscillation amplitude and frequency are A = 0.428 and u> = 1.303, the first Fourier amplitude (corresponding to frequency u>) is Ai = 0.42. Due to the cubic nonlinearity in the restoring forcé, in turn, the next significant amplitude corresponds to the third-harmonic component (frequency 3w), A3, = 0.0076. Higher-harmonic amplitudes are even smaller. The first correction to harmonio motion, thus, is almost two orders of magnitude weaker than the main contribution, which reasonably justifies our analytical approxi-mation. On the other hand, this modest contribution of higher-harmonic components to the overall motion markedly contrasts with the sizable phe-nomenology studied in section II., which is also a direct consequence of nonlinearity.

V. Conclusions

We have analyzed the dynamics of a mechanical oscillator whose periodic motion is sustained by a feedback forcé proportional to the oscillation veloc-ity. The key ingredient that makes oscillations sta-ble is a nonlinear dependence of damping with the oscillation amplitude, such that energy dissipation increases or decreases when the amplitude respec-tively grows or drops. This kind of nonlinearity, together with a cubic component in the restoring forcé, has been experimentally observed to occur in c-c beam micromechanical oscillators [6], which can therefore exhibit self-sustained motion under the action of linear velocity feedback.

Our emphasis was put on the effect of a time delay in the feedback forcé, assumed to originate in the electric circuit that reads, conditions, and rein-jects the oscillation signal. The most significant consequence of this ingredient is that oscillation can be suppressed if the delay is large enough. It is well known that differential equations with time-delayed terms can exhibit either stationary oscillatory solutions or fixed rest points, depending on the delay [10]. Also, oscillation suppression (or "death") due to delays has been reported to occur in a variety of dynamical systems, such as synchronized limit-cycle oscillators [11,12]. Here, this same occurrence has been characterized for a nonlinear mechanical system that can become relevant for the design of micromechanical time-keeping devices.

In connection with possible applications of this phenomenology, it is worth mentioning that classical-mechanical models such as Eq. (1) pro-vide a suitable starting point for the description of micro- and nanomechanical machines. As length scales become smaller, however, the effects of ther-mal fluctuations and electrical noise cannot be fur-ther ignored [13, 14]. From the viewpoint of our model, therefore, an important step forward would be to include noise in the theoretical analysis.

 

Acknowledgements - This project has been supported by Agencia Nacional de Promoción Científica y Tecnológica, Argentina, through grant PICT2014-1611. Fruitful collaboration with D. Antonio, S. Arroyo, Changyao Chen, D. Czaplewski, J. Guest, D. López, and F. Mangussi, as well as discussions with S. Risau Gusmn, are gratefully acknowledged.

1. B Yurke, D S Greywall, A N Pargellis, P A Busch, Theory of amplifier-noise evasion in an oscillator employing a nonlinear resonator, Phys. Rev. A 51, 4211 (1995).         [ Links ]

2. D Bishop, P Gammel, R Giles, The little machines that are making it big, Phys. Today 54, 38 (2001).         [ Links ]

3 K L Ekinci, M L Roukes, Nanoelectromechan-ical systems, Rev. Sci. Instrum. 76, 061101 (2005).         [ Links ]

4 D Antonio, D H Zanette, D L´opez, Frequency stabilization in nonlinear micromechanical oscillators, Nat. Commun. 3, 806 (2012).         [ Links ]

5 D Antonio, D A Czaplewski, J R Guest, D L´opez, S I Arroyo, D H Zanette, Nonlinearity-induced synchronization enhancement in mi-cromechanical oscillators, Phys. Rev. Lett. 114, 034103 (2015).         [ Links ]

6 Changyao Chen, D H Zanette, J R Guest, D A Czaplewski, D L´opez, Self-sustained microme-chanical oscillator with linear feedback, Phys. Rev. Lett. 117, 017203 (2016).         [ Links ]

7 A H Nayfeh, D T Mook, Nonlinear Oscilla-tions, Wiley, New York (2008).         [ Links ]

8 H B Peng, C W Chang, S Aloni, T D Yuzvin-sky, A Zettl, Ultrahigh frequency nanotube res-onators, Phys. Rev. Lett. 97, 087203 (2006).         [ Links ]

9 S I Arroyo, D H Zanette, Duffing revisited: Phase-shift control and internal resonance in self-sustained oscillators, Eur. Phys. J. B 89, 12 (2016).         [ Links ]

10 T Erneux, Applied Delay Differential Equations, Springer, New York (2009).         [ Links ]

11 D V Ramana Reddy, A Sen, G L Johnston, Time delay induced death in coupled limit cycle oscillators, Phys. Rev. Lett. 80, 5109 (1998).         [ Links ]

12 S H Strogatz, Death by delay, Nature 394, 316 (1998).         [ Links ]

13 A N Cleland, M L Roukes, Noise processes in nanomechanical resonators, J. Appl. Phys. 92, 2758 (2002).         [ Links ]

14 P Ward, A Duwel, Oscillator phase noise: sys-tematic construction of an analytical mode en-compassing nonlinearity, IEEE Trans. Ultrason. Ferroelectr. Freq. Control 58, 195 (2011).         [ Links ]